Functional Beamforming (FBF)

How does Functional Beamforming work?

A point spread function with a large width of the main lobe and low dynamic allows dominant sound sources to cover weaker ones which may lead to a distorted acoustic map. FBF $B_{\nu}(\vec{x},\omega)$ is an extension of delay-and-sum-beamforming in the frequency domain (FDBF) and provides a sharper main lobe and larger dynamics without increasing calculation time significantly. Like orthogonal beamforming (OBF), it is based on the decomposition of the cross spectral matrix $C$ into the diagonal matrix $\Lambda$ of its eigenvalues and the matrix $V$containing its corresponding eigenvectors: $$B_{\nu}(\vec{x},\omega)=\left(g^H(\vec{x},\omega)C^{\frac{1}{\nu}}g(\vec{x},\omega)\right)^{\nu},\qquad C^{\frac{1}{\nu}}=V\Lambda^{\frac{1}{\nu}}V^H.$$

In doing so, $g(\vec{x},\omega)$ are the so called steering vectors to the possible source locations $\vec{x}$and $V^H$ is the transposed-conjugated matrix of eigenvectors. The variable exponent $\nu \geq 1$ specifies the degree of FBF. The higher its value, the narrower is the main lobe. Furthermore, the dynamic range increases proportionally to the exponent $\nu$.

No significant improvements can be noticed, however, above a certain value of the exponent $\nu$. Therefore, $\nu$ is limited to $1\leq\nu\leq 50$ in NoiseImage. Using an exponent of $\nu=1$ corresponds to the results of FDBF. When using FBF, a combination with OBF can lead to a better understanding of the source characteristics. However, if some sources are coherent, FBF should not be used since side lobes could be interpreted as sound sources.

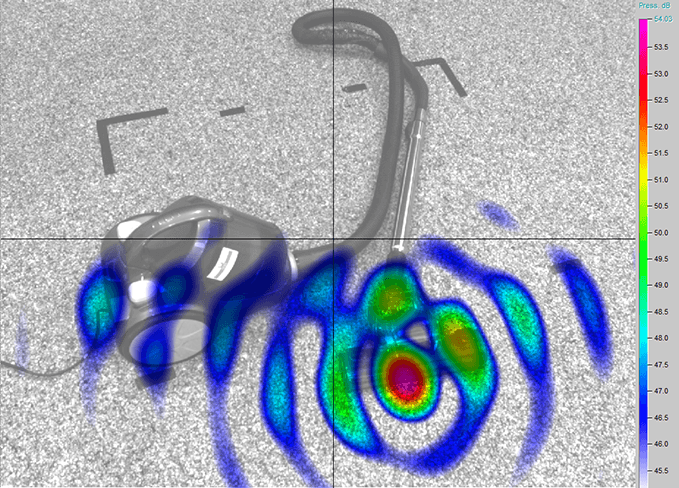
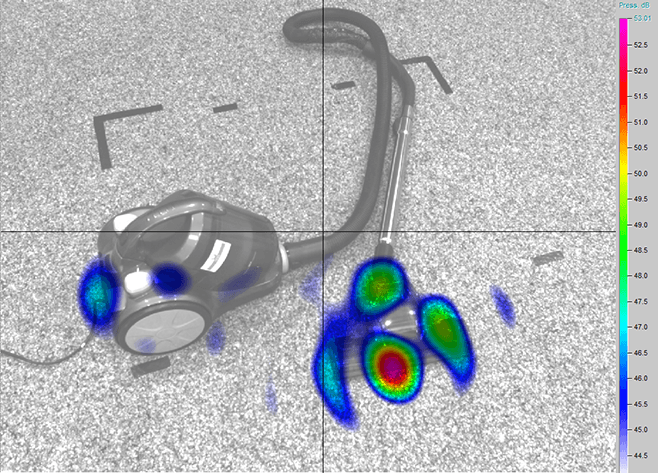
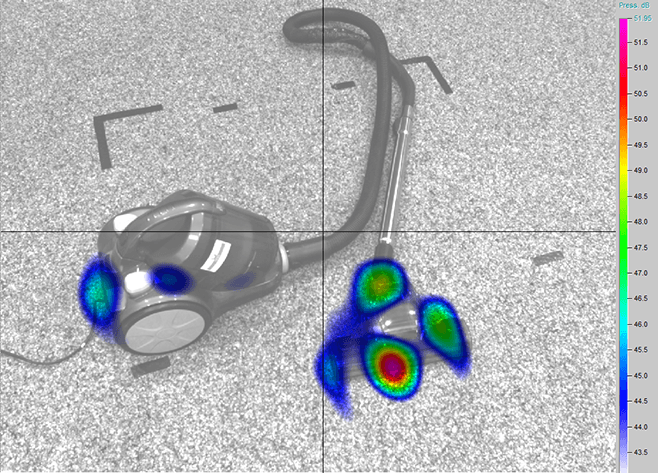
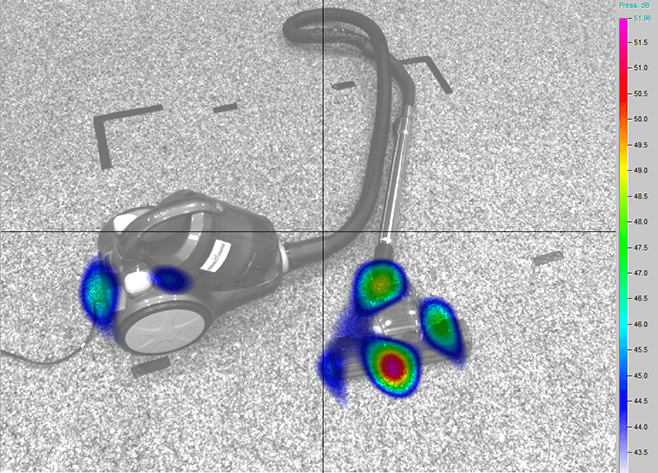
Application Example: Vacuum Cleaner

In the example of the vacuum cleaner shown below, it is obvious that FBF does not change the source strength considerably compared to FDBF and it also decreases the width of sound sources at the brush. If $\nu\geq 5$, however, there is no further improvement of source representation to be noticed. All images show results within the frequency range from 5.44 kHz to 6.14 kHz.

Fig. 1: FDBF - corresponding to FBF with $\nu$=1